
Photo by YouTube/Mind Your Decisions
In a 2020 video, mathematician Presh Talwalker of the YouTube channel Mind Your Decisions poses a particularly tough math problem.
It’s a letter problem, meaning each of the letters in the equation corresponds to a single digit.
Talwalker says teachers in Taiwan gave this problem to 9-year-olds, who understandably couldn’t solve it—and neither could the teachers. So let’s break it down:

Photo by Mind Your Decisions/Presh Talwalker
Before we begin...
I can only walk you through how my mind puzzled out the math here. But what stood out to me is that
E is the last value on the first line and in the solution. There aren’t a lot of digits you multiply by that result in themselves again, which meant it’s almost definitely a 5. Let’s substitute that and see what’s next. If it doesn't work, we can try something else.

Photo by Caroline Delbert
This looks good to me. Now, we have another logical hook to unlock the next step. 555555 has a set number of factors, and we only have to test if it’s divisible by any of the positive integers less than 10. Obviously it’s divisible by 5, but we know A can't also be 5—all the “lettered” digits are different. It’s divisible by 3, but not 9.
There’s no five-digit number we can multiply by 3 to get a
six
-digit number starting with 5. It’s not divisible by any even numbers. The highest value that A could have is 7. Is 555555 divisible by 7? Contextually, I feel it must be.
Some of the division rules for small integers are really easy. The one for 7, however, is not easy. Honestly, in this case, I’d just actually divide the number by 7, which is what I did in reality. But let’s also walk through the division rule for 7, because it’s interesting in itself, speaking of algorithmic thinking.
Here’s that rule, explained by a site called Byju’s :
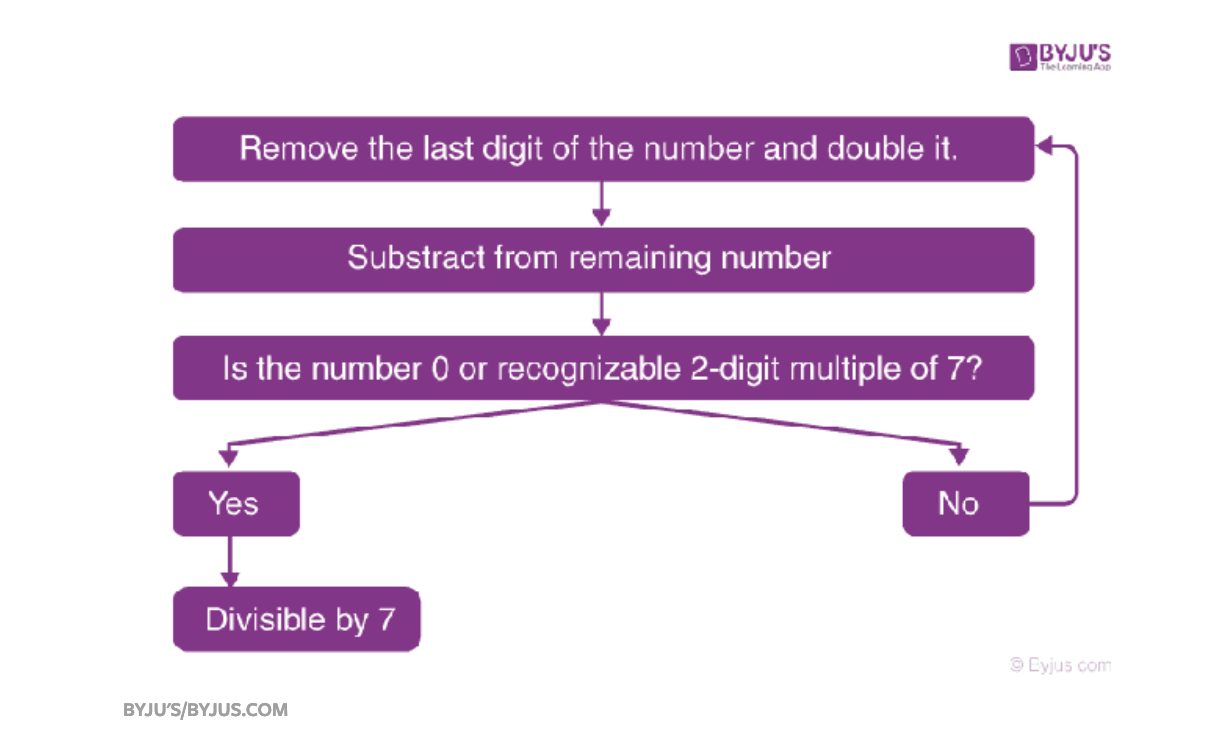
So let’s process 555555 using the rule, iterating from our original number of 555555 all the way down to, hopefully, a two-digit number that’s divisible by 7.

Photo by Caroline Delbert
When we get down to a result of 42, we know the number 555555 is divisible by 7. Now we can just do the division and get the result: 79365.
When we multiply this through to check our work, it’s the right answer.

Photo by Caroline Delbert
Talwalker solves the problem in a totally different way. Watch his great video and see that full solution, because he’s really doing the math in a comprehensive way:
My way, informed by decades of puzzle solving, involves narrowing the possibilities using logic and then checking my educated guess. Maybe you did something totally different.