
Photo by eugenesergeev / Getty Images.
In the 1960s, the charismatic physicist Geoffrey Chew espoused a radical vision of the universe, and with it, a new way of doing physics. Theorists of the era were struggling to find order in an unruly zoo of newfound particles. They wanted to know which ones were the fundamental building blocks of nature and which were composites. But Chew, a professor at the University of California, Berkeley, argued against such a distinction. “Nature is as it is because this is the only possible nature consistent with itself,” he wrote at the time. He believed he could deduce nature’s laws solely from the demand that they be self-consistent.
Scientists since Democritus had taken a reductionist approach to understanding the universe, viewing everything in it as being built from some kind of fundamental stuff that cannot be further explained. But Chew’s vision of a self-determining universe required that all particles be equally composite and fundamental. He conjectured that each particle is composed of other particles, and those others are held together by exchanging the first particle in a process that conveys a force. Thus, particles’ properties are generated by self-consistent feedback loops. Particles, Chew said, “pull themselves up by their own bootstraps.”
Chew’s approach, known as the bootstrap philosophy, the bootstrap method, or simply “the bootstrap,” came without an operating manual. The point was to apply whatever general principles and consistency conditions were at hand to infer what the properties of particles (and therefore all of nature) simply had to be. An early triumph in which Chew’s students used the bootstrap to predict the mass of the rho meson — a particle made of pions that are held together by exchanging rho mesons — won many converts.
But the rho meson turned out to be something of a special case, and the bootstrap method soon lost momentum. A competing theory cast particles such as protons and neutrons as composites of fundamental particles called quarks. This theory of quark interactions, called quantum chromodynamics, better matched experimental data and soon became one of the three pillars of the reigning Standard Model of particle physics.
But the properties of individual quarks seemed arbitrary, and in another universe they might have been different. Physicists were forced to recognize that the set of particles that happen to populate the universe do not reflect the only possible consistent theory of nature. Rather, an endless variety of possible particles can be imagined interacting in any number of spatial dimensions, each situation described by its own “quantum field theory.”
The bootstrap languished for decades at the bottom of the physics toolkit. But recently the field has been re-energized as physicists have discovered novel bootstrap techniques that appear to solve many problems. While consistency conditions still aren’t much help for sorting out complicated nuclear particle dynamics, the bootstrap is proving to be a powerful tool for understanding more symmetric, perfect theories that, according to experts, serve as “signposts” or “building blocks” in the space of all possible quantum field theories.
As the new generation of bootstrappers explores this abstract theory space, they seem to be verifying the vision that Chew, now 92 and long retired, laid out half a century ago — but they’re doing it in an unexpected way. Their findings indicate that the set of all quantum field theories forms a unique mathematical structure, one that does indeed pull itself up by its own bootstraps, which means it can be understood on its own terms.
As physicists use the bootstrap to explore the geometry of this theory space, they are pinpointing the roots of “universality,” a remarkable phenomenon in which identical behaviors emerge in materials as different as magnets and water. They are also discovering general features of quantum gravity theories, with apparent implications for the quantum origin of gravity in our own universe and the origin of space-time itself. As leading practitioners David Poland of Yale University and David Simmons-Duffin of the Institute for Advanced Study in Princeton, New Jersey, wrote in a 2016 article, “It is an exciting time to be bootstrapping.”
Bespoke Bootstrap
The bootstrap is technically a method for computing “correlation functions” — formulas that encode the relationships between the particles described by a quantum field theory. Consider a chunk of iron. The correlation functions of this system express the likelihood that iron atoms will be magnetically oriented in the same direction, as a function of the distances between them. The two-point correlation function gives you the likelihood that any two atoms will be aligned, the three-point correlation function encodes correlations between any three atoms, and so on. These functions tell you essentially everything about the iron chunk. But they involve infinitely many terms riddled with unknown exponents and coefficients. They are, in general, onerous to compute. The bootstrap approach is to try to constrain what the terms of the functions can possibly be in hopes of solving for the unknown variables. Most of the time, this doesn’t get you far. But in special cases, as the theoretical physicist Alexander Polyakov began to figure out in 1970, the bootstrap takes you all the way.
Polyakov, then at the Landau Institute for Theoretical Physics in Russia, was drawn to these special cases by the mystery of universality. As condensed matter physicists were just discovering, when materials that are completely different at the microscopic level are tuned to the critical points at which they undergo phase transitions, they suddenly exhibit the same behaviors and can be described by the exact same handful of numbers. Heat iron to the critical temperature where it ceases to be magnetized, for instance, and the correlations between its atoms are defined by the same “critical exponents” that characterize water at the critical point where its liquid and vapor phases meet. These critical exponents are clearly independent of either material’s microscopic details, arising instead from something that both systems, and others in their “universality class,” have in common. Polyakov and other researchers wanted to find the universal laws connecting these systems. “And the goal, the holy grail of all that, was these numbers,” he said: Researchers wished to be able to calculate the critical exponents from scratch.
What materials at critical points have in common, Polyakov realized, is their symmetries: the set of geometric transformations that leave these systems unchanged. He conjectured that critical materials respect a group of symmetries called “conformal symmetries,” including, most importantly, scale symmetry. Zoom in or out on, say, iron at its critical point, and you always see the same pattern: Patches of atoms oriented with north pointing up are surrounded by patches of atoms pointing downward; these in turn are inside larger patches of up-facing atoms, and so on at all scales of magnification. Scale symmetry means there are no absolute notions of “near” and “far” in conformal systems; if you flip one of the iron atoms, the effect is felt everywhere. “The whole thing organizes as some very strongly correlated medium,” Polyakov explained.
The world at large is obviously not conformal. The existence of quarks and other elementary particles “breaks” scale symmetry by introducing fundamental mass and distance scales into nature, against which other masses and lengths can be measured. Consequently, planets, composed of hordes of particles, are much heavier and bigger than we are, and we are much larger than atoms, which are giants next to quarks. Symmetry-breaking makes nature hierarchical and injects arbitrary variables into its correlation functions — the qualities that sapped Chew’s bootstrap method of its power.
But conformal systems, described by “conformal field theories” (CFTs), are uniform all the way up and down, and this, Polyakov discovered, makes them highly amenable to a bootstrap approach. In a magnet at its critical point, for instance, scale symmetry constrains the two-point correlation function by requiring that it must stay the same when you rescale the distance between the two points. Another conformal symmetry says the three-point function must not change when you invert the three distances involved. In a landmark 1983 paper known simply as “BPZ,” Alexander Belavin, Polyakov and Alexander Zamolodchikov showed that there are an infinite number of conformal symmetries in two spatial dimensions that could be used to constrain the correlation functions of two-dimensional conformal field theories. The authors exploited these symmetries to solve for the critical exponents of a famous CFT called the 2-D Ising model — essentially the theory of a flat magnet. The “conformal bootstrap,” BPZ’s bespoke procedure for exploiting conformal symmetries, shot to fame.
Far fewer conformal symmetries exist in three dimensions or higher, however. Polyakov could write down a “bootstrap equation” for 3-D CFTs — essentially, an equation saying that one way of writing the four-correlation function of, say, a real magnet must equal another — but the equation was too difficult to solve.
“I basically started doing other things,” said Polyakov, who went on to make seminal contributions to string theory and is now a professor at Princeton University. The conformal bootstrap, like the original bootstrap more than a decade earlier, fell into disuse. The lull lasted until 2008, when a group of researchers discovered a powerful trick for approximating solutions to Polyakov’s bootstrap equation for CFTs with three or more dimensions. “Frankly, I didn’t expect this, and I thought originally that there is some mistake there,” Polyakov said. “It seemed to me that the information put into the equations is too little to get such results.”
Surprise Kinks
In 2008, the Large Hadron Collider was about to begin searching for the Higgs boson, an elementary particle whose associated field imbues other particles with mass. Theorists Riccardo Rattazzi in Switzerland, Vyacheslav Rychkov in Italy and their collaborators wanted to see whether there might be a conformal field theory that is responsible for the mass-giving instead of the Higgs. They wrote down a bootstrap equation that such a theory would have to satisfy. Because this was a four-dimensional conformal field theory, describing a hypothetical quantum field in a universe with four space-time dimensions, the bootstrap equation was too complex to solve. But the researchers found a way to put bounds on the possible properties of that theory. In the end, they concluded that no such CFT existed (and indeed, the LHC found the Higgs boson in 2012). But their new bootstrap trick opened up a gold mine.
Their trick was to translate the constraints on the bootstrap equation into a geometry problem. Imagine the four points of the four-point correlation function (which encodes virtually everything about a CFT) as corners of a rectangle; the bootstrap equation says that if you perturb a conformal system at corners one and two and measure the effects at corners three and four, or you tickle the system at one and three and measure at two and four, the same correlation function holds in both cases. Both ways of writing the function involve infinite series of terms; their equivalence means that the first infinite series minus the second equals zero. To find out which terms satisfy this constraint, Rattazzi, Rychkov and company called upon another consistency condition called “unitarity,” which demands that all the terms in the equation must have positive coefficients. This enabled them to treat the terms as vectors, or little arrows that extend in an infinite number of directions from a central point. And if a plane could be found such that, in a finite subset of dimensions, all the vectors point to one side of the plane, then there’s an imbalance; this particular set of terms cannot sum to zero, and does not represent a solution to the bootstrap equation.
Physicists developed algorithms that allowed them to search for such planes and bound the space of viable CFTs to extremely high accuracy. The simplest version of the procedure generates “exclusion plots” where two curves meet at a point known as a “kink.” The plots rule out CFTs with critical exponents that lie outside the area bounded by the curves.
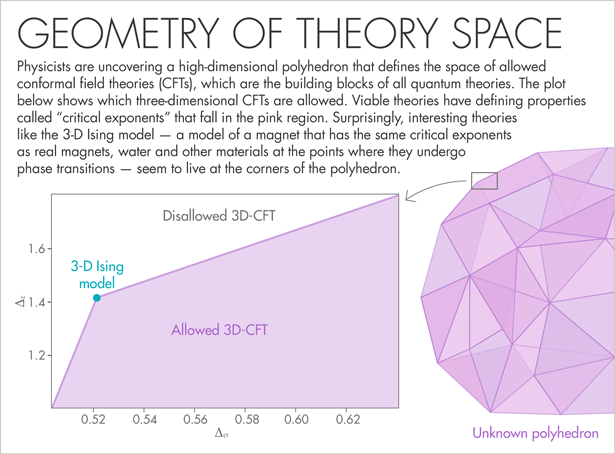
Credit: Lucy Reading-Ikkanda/Quanta Magazine. Graph adapted from Nature Physics 537 (2016)
The discovery was so unexpected that Polyakov initially didn’t believe it. His suspicion, shared by others, was that “maybe this happens because there is some hidden symmetry that we didn’t find yet.”
“Everyone is excited because these kinks are unexpected and interesting, and they tell you where interesting theories live,” said Nima Arkani-Hamed, a professor of physics at the Institute for Advanced Study. “It could be reflecting a polyhedral structure of the space of allowed conformal field theories, with interesting theories living not in the interior or some random place, but living at the corners.” Other researchers agreed that this is what the plots suggest. Arkani-Hamed speculates that the polyhedron is related to, or might even encompass, the “amplituhedron,” a geometric object that he and a collaborator discovered in 2013 that encodes the probabilities of different particle collision outcomes — specific examples of correlation functions.
Researchers are pushing in all directions. Some are applying the bootstrap to get a handle on an especially symmetric “superconformal” field theory known as the (2,0) theory, which plays a role in string theory and is conjectured to exist in six dimensions. But Simmons-Duffin explained that the effort to explore CFTs will take physicists beyond these special theories. More general quantum field theories like quantum chromodynamics can be derived by starting with a CFT and “flowing” its properties using a mathematical procedure called the renormalization group. “CFTs are kind of like signposts in the landscape of quantum field theories, and renormalization-group flows are like the roads,” Simmons-Duffin said. “So you’ve got to first understand the signposts, and then you can try to describe the roads between them, and in that way you can kind of make a map of the space of theories.”
Tom Hartman, a bootstrapper at Cornell University, said mapping out the space of quantum field theories is the “grand goal of the bootstrap program.” The CFT plots, he said, “are some very fuzzy version of that ultimate map.”
Uncovering the polyhedral structure representing all possible quantum field theories would, in a sense, unify quark interactions, magnets and all observed and imagined phenomena in a single, inevitable structure — a sort of 21st-century version of Geoffrey Chew’s “only possible nature consistent with itself.” But as Hartman, Simmons-Duffin and scores of other researchers around the world pursue this abstraction, they are also using the bootstrap to exploit a direct connection between CFTs and the theories many physicists care about most. “Exploring possible conformal field theories is also exploring possible theories of quantum gravity,” Hartman said.
Bootstrapping Quantum Gravity
The conformal bootstrap is turning out to be a power tool for quantum gravity research. In a 1997 paper that is now one of the most highly cited in physics history, the Argentinian-American theorist Juan Maldacena demonstrated a mathematical equivalence between a CFT and a gravitational space-time environment with at least one extra spatial dimension. Maldacena’s duality, called the “AdS/CFT correspondence,” tied the CFT to a corresponding “anti-de Sitter space,” which, with its extra dimension, pops out of the conformal system like a hologram. AdS space has a fish-eye geometry different from the geometry of space-time in our own universe, and yet gravity there works in much the same way as it does here. Both geometries, for instance, give rise to black holes — paradoxical objects that are so dense that nothing inside them can escape their gravity.
Existing theories do not apply inside black holes; if you try to combine quantum theory there with Albert Einstein’s theory of gravity (which casts gravity as curves in the space-time fabric), paradoxes arise. One major question is how black holes manage to preserve quantum information, even as Einstein’s theory says they evaporate. Solving this paradox requires physicists to find a quantum theory of gravity — a more fundamental conceptualization from which the space-time picture emerges at low energies, such as outside black holes. “The amazing thing about AdS/CFT is, it gives a working example of quantum gravity where everything is well-defined and all we have to do is study it and find answers to these paradoxes,” Simmons-Duffin said.
If the AdS/CFT correspondence provides theoretical physicists with a microscope onto quantum gravity theories, the conformal bootstrap has allowed them to switch on the microscope light. In 2009, theorists used the bootstrap to find evidence that every CFT meeting certain conditions has an approximate dual gravitational theory in AdS space. They’ve since been working out a precise dictionary to translate between critical exponents and other properties of CFTs and equivalent features of the AdS-space hologram.
Over the past year, bootstrappers like Hartman and Jared Kaplan of Johns Hopkins University have made quick progress in understanding how black holes work in these fish-eye universes, and in particular, how information gets preserved during black hole evaporation. This could significantly impact the understanding of the quantum nature of gravity and space-time in our own universe. “If I have some small black hole, it doesn’t care whether it’s in AdS space; it’s small compared to the size of the curvature,” Kaplan explained. “So if you can resolve these conceptual issues in AdS space, then it seems very plausible that the same resolution applies in cosmology.”
It’s far from clear whether our own universe holographically emerges from a conformal field theory in the way that AdS universes do, or if this is even the right way to think about it. The hope is that, by bootstrapping their way around the unifying geometric structure of possible physical realities, physicists will get a better sense of where our universe fits in the grand scheme of things — and what that grand scheme is. Polyakov is buoyed by the recent discoveries about the geometry of the theory space. “There are a lot of miracles happening,” he said. “And probably, we will know why.”
Natalie Wolchover is a senior writer and editor at Quanta Magazine covering the physical sciences.